Comparative Analysis of Ridge, Bridge and Lasso Regression Models In the Presence of Multicollinearity

Abstract

Aims: This research work investigated the best regression technique in handling multicollinearity using the Ridge, Least Absolute Shrinkage and Selection Operator (LASSO) and Bridge regression models in comparison to Analysis and Prediction.

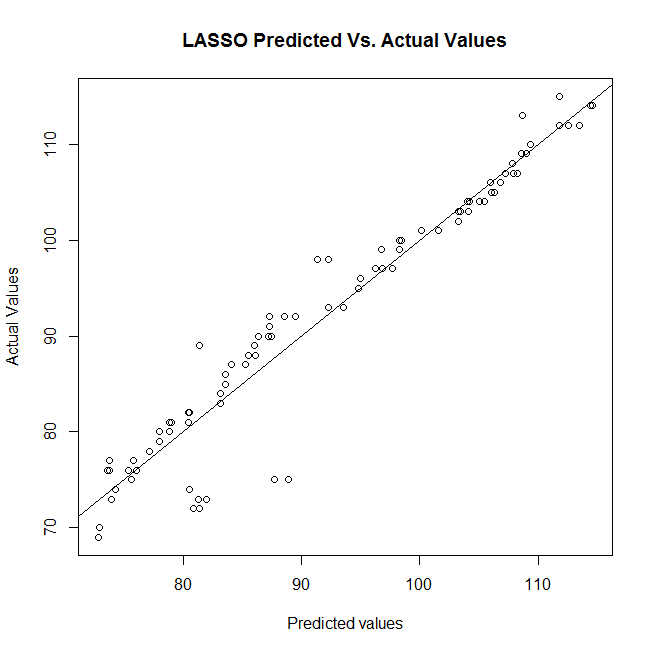
Study Design: Two sets of secondary data on Body Size and Heart Rate gotten from the Lulu Briggs Health Center, University of Port Harcourt were used for comparison for model fit and in handling multicollinearity between the regression techniques. Tables were used to present Comparisons made using MSE, RMSE, VIF, AIC and BIC for efficiency. Scatter plots were employed to show fitted regression models. R Software was used to perform data analysis.

Methodology: The data were tested for the presence of Multicollinearity using VIF respectively, before proceeding to apply Ridge, LASSO and Bridge regression techniques to solve the problem of multicollinearity. Then comparison was made in analysis and prediction between the regression techniques.               

Results: The results from the study show that, for analysis on body size, we found that none of the Regression Techniques handled the problem of multicollinearity, even though the degree of multicollinearity present in the data set reduces, with VIF values of 11.36762 for Ridge, 10.8042 for LASSO, and Bridge which are 10.95578, 11.24945, 12.22628 and 12.14645 respectively. For Heart Rate analysis, we see that all the regularized regression techniques handled the problem of multicollinearity. The results show that the Bridge regression technique performed better with a VIF of 1.744461 when